research topic on mathematics education

Trends in mathematics education and insights from a meta-review and bibliometric analysis of review studies

• Original Paper
• Open access
• Published: 15 May 2024
• Volume 56 , pages 165–188, ( 2024 )

Cite this article

You have full access to this open access article

• Mustafa Cevikbas   ORCID: orcid.org/0000-0002-7844-4707 1 ,
• Gabriele Kaiser 2 , 3 &
• Stanislaw Schukajlow 4  

4566 Accesses

3 Altmetric

Explore all metrics

Review studies are vital for advancing knowledge in many scientific fields, including mathematics education, amid burgeoning publications. Based on an extensive consideration of existing review typologies, we conducted a meta-review and bibliometric analysis to provide a comprehensive overview of and deeper insights into review studies within mathematics education. After searching Web of Science, we identified 259 review studies, revealing a significant increase in such studies over the last five years. Systematic reviews were the most prevalent type, followed by meta-analyses, generic literature reviews, and scoping reviews. On average, the review studies had a sample size of 99, with the Preferred Reporting Items for Systematic Reviews and Meta-Analysis (PRISMA) guidelines commonly employed. Despite certain studies offering nuanced distinctions among review types, ambiguity persisted. Only about a quarter of the studies explicitly reported employing specific theoretical frameworks (particularly, technology, knowledge, and competence models). Co-authored publications were most common within American institutions and the leading countries are the United States, Germany, China, Australia, and England in publishing most review studies. Educational review journals, educational psychology journals, special education journals, educational technology journals, and mathematics education journals provided platforms for review studies, and prominent research topics included digital technologies, teacher education, mathematics achievement, and learning disabilities. In this study, we synthesised a range of reviews to facilitate readers’ comprehension of conceptual congruities and disparities across various review types, as well as to track current research trends. The results suggest that there is a need for discipline-specific standards and guidelines for different types of mathematics education reviews, which may lead to more high-quality review studies to enhance progress in mathematics education.

Similar content being viewed by others

Looking Back and Taking Stock: Reflections on the MERGA Research Review 2012–2015

Educational Studies in Mathematics: Shaping the Field

Adult education in mathematics and numeracy: a scoping review of recent research

Avoid common mistakes on your manuscript.

1 Introduction

Comprehensive literature reviews serve as foundational pillars for advancing scholarly discourse, offering critical insights into existing research and shaping future inquiries across disciplines. In the realm of academic writing, spanning from journal articles to dissertations, literature reviews are highly regarded for their capacity to synthesize knowledge, identify gaps, and provide a cohesive framework for understanding complex topics (Boote & Beile, 2005 ). Moreover, reviews play a significant role in academia by setting new research agendas and informing decision-making processes in practice, policy, and society (Kunisch et al., 2023 ).

As empirical and theoretical research burgeons in diverse fields, the need for literature review studies has become even more pronounced, facilitating a deeper understanding of specific research areas or themes (Hart, 2018 ; Nane et al., 2023 ). Additional factors contributing to the popularity of review studies in recent years include the rise of specialized review journals (Kunisch et al., 2023 ), challenges associated with conducting various types of empirical studies during the prolonged COVID-19 crisis (Cevikbas & Kaiser, 2023 ), and a competitive research climate wherein factors such as impact factors and citations hold significant weight (Ketcham & Crawford, 2007 ). Review studies are particularly attractive as they often garner a substantial number of citations, thereby enhancing researchers’ visibility and scholarly impact (Grant & Booth, 2009 ; Taherdoost, 2023 ).

The importance of review studies has been duly acknowledged in mathematics education, as evidenced by the inclusion of review papers in thematically oriented special issues of journals such as ZDM– Mathematics Education (Kaiser & Schukajlow, 2024 ), which has been originally founded as review journal. Several upcoming or already published special issues of ZDM– Mathematics Education , which emphasise ‘reviews on important themes in mathematics education’, highlight the importance of review studies as valuable contributions to the field.

The proliferation of literature reviews has increased interest in developing typologies to categorise them and understand different literature review approaches (Grant & Booth, 2009 ; Paré et al., 2015 ; Schryen & Sperling, 2023 ). Despite its significance, there remains a notable lack of research aimed at comprehensively understanding review studies within the field of mathematics education from a meta-perspective. In response to this gap, we conducted a systematic meta-review with the aim of providing an overview of different types of review studies in mathematics education over the past few decades and consolidating insights from multiple high-level review studies (Becker & Oxman, 2008 ; Schryen & Sperling, 2023 ). Meta-reviews offer concise yet comprehensive synopses and curated lists of pertinent reviews, adeptly addressing the perennial challenge of balancing thorough coverage with focused specificity (Grant & Booth, 2009 ).

In addition, we applied bibliometric analysis as a valuable tool for identifying research trends, progress, reliable sources, and future directions within the field. The bibliometric analysis aids in identifying hot research topics and trends (Song et al., 2019 ), assessing progress, identifying reliable sources, recognising major contributors, and predicting future research success (Geng et al., 2017 ). Furthermore, it helps researchers to pinpoint potential topics, suitable institutions for cooperation, and potential scholars for scientific collaboration (Martínez et al., 2015 ). By combining a meta-review and bibliometric analysis, we aim to offer a comprehensive overview of and deeper insights into state-of-the-art review studies within mathematics education.

Specifically, we seek to understand how the distribution and development of literature review studies in mathematics education have evolved over the years, examining factors such as publication years, publishers, review types, sample sizes, and the use of theoretical or conceptual frameworks. Additionally, we aim to assess adherence to review study guidelines and protocols, providing insights into the rigor and quality of research methodologies employed, particularly in light of the lack of clear guidance on producing rigorous and impactful literature reviews (Kunisch et al., 2023 ).

Furthermore, we endeavour to identify authors who have made contribution to the field of mathematics education through review studies, as well as those whose work is most frequently cited. We also identify co-authorship network analysis as understanding research networks allows researchers to identify potential collaborators and build partnerships with other scholars in various countries. Collaborative research endeavours can lead to enhanced research outcomes, broader dissemination of findings, and increased opportunities for funding and professional development. It can also highlight interdisciplinary connections and collaborations within and across fields, leading to innovative approaches and solutions to complex research questions (RQs) that transcend disciplinary boundaries.

Moreover, we analysed the distribution of common keywords across review studies, identifying focal subjects and thematic areas prevalent in mathematics education research. This analysis can provide valuable insights into key topics and trends shaping the field, guiding future research directions and priorities.

Lastly, we identified the most cited review papers in mathematics education and the journals in which they have been published, recognizing seminal works and influential publications that have contributed to the advancement of the field.

Overall, in light of the preceding discourse, we addressed the following RQs to uncover the characteristics of review studies, identify research trends, and delineate future research directions in mathematics education:

How can the distribution and development of review studies in mathematics education over time be characterised according to the number of manuscripts, publishers, review types, sample sizes, the use of theoretical or conceptual frameworks, and adherence to review study guidelines and protocols?

Which authors have contributed the largest number of review studies in mathematics education, and which authors’ review papers are most frequently cited in the literature?

From which countries are the authors of the review studies in mathematics education?

Which author keywords can be identified in the review studies in mathematics education, how are these keywords distributed across the analysed review studies, and which focal topics do these keywords indicate?

What are the most cited review papers in mathematics education, and in which journals have they been published?

2 Literature review studies and review typologies– background information

In this chapter, we provide a thorough analysis of different typologies for review studies, as we seek to elucidate the primary characteristics of various review studies conducted within mathematics education (Sect.  2.1 ). This effort led to the identification of 28 review types presented in Table  1 , which were used in the current study’s literature search processes to access existing review studies and the analysis of identified studies in the field of mathematics education. Furthermore, we discuss the advancement of guidelines and protocols, highlighting their role in shaping the conduct of review studies (Sect. 2.2). Finally, we conclude the chapter by underscoring the importance and potential impact of meta-reviews and bibliometric analyses in the context of mathematics education (Sect. 2.3).

2.1 Literature review typologies

Researchers have defined and emphasized different review types with distinct features, objectives, and methodologies. To address the challenge of ambiguous review categorisations, we conducted an extensive search and analysis of the literature on Web of Science (WoS) using the search strings ‘typology of reviews’ and ‘taxonomy of reviews’ to search the titles of studies. We focused particularly on influential theoretical, conceptual, and review papers discussing the taxonomy and typology of review studies and recent advances driven by scholars across diverse fields.

2.1.1 Seminal work by Grant and Booth ( 2009 ) on the discourse of literature review typologies

The categorisation of literature reviews has been profoundly influenced by the seminal work of Grant and Booth ( 2009 ), on which typologies of literature reviews are often based. Their paper garnered significant attention, with over 10,304 citations as of 20 April 2024 according to Google Scholar. Originally in the field of health information theory and practice, these authors founded their work on earlier approaches, notably Cochrane’s ( 1979 ) approach. Grant and Booth ( 2009 ) claimed that the developed typology could standardise the diverse terminology used. They distinguished 14 review types, which we summarise below, highlighting the main scope and search methodologies (Grant & Booth, 2009 , pp. 94–95):

A critical review ‘goes beyond mere description of identified articles and includes a degree of analysis and conceptual innovation’; no formalised or systematic approach is required because the aim of such a review is ‘to identify conceptual contributions to embody existing or derive new theory’.

A generic literature review incorporates ‘published materials that provide examination of recent of current literature’; comprehensive searching may or may not be necessary.

A mapping review/systematic mapping is used to ‘categorize existing literature’ and identify gaps in the research literature. The completeness of a search is important, but no formal quality assessment is needed.

A meta-analysis is a ‘technique that statistically combines the results of quantitative studies to provide a more precise effect of the results’; a comprehensive search is conducted based on the inclusion and exclusion criteria.

A mixed-studies review/mixed-methods review incorporates ‘a combination of review approaches, for example combining quantitative with qualitative research… and requires a very sensitive search’.

An overview is a generic term describing a ‘summary of the… literature that attempts to survey the literature and describe its characteristics’; it may or may not include comprehensive searching and quality assessment.

A qualitative systematic review/qualitative evidence synthesis is a ‘method for integrating or comparing the findings from qualitative studies’, and it may involve selective sampling.

A rapid review comprises an ‘assessment of what is already known about a policy or practice issue, by using systematic review methods to search and critically appraise existing research’; a characteristic of such a review is that the ‘completeness of searching is determined by time constraints’.

A scoping review is a ‘preliminary assessment of the potential size and scope of available research literature’, with the ‘completeness of searching determined by time/scope constraints’.

A state-of-the-art review ‘tend[s] to address more current matters in contrast to other combined retrospective and current approaches’ and ‘aims for comprehensive searching of current literature’.

A systematic review ‘seeks to systematically search for, appraise and synthesise research evidence’ and should be comprehensive and based on inclusion/exclusion criteria.

A systematic search and review ‘combines [the] strengths of critical review with a comprehensive search process’, typically addressing broad questions to produce ‘best evidence synthesis’ based on ‘exhaustive, comprehensive searching’.

A systematised review ‘include[s] elements of systematic review process while stopping short of systematic review’, ‘typically conducted as postgraduate student assignment’; it ‘may or may not include comprehensive searching’.

An umbrella review ‘specifically refers to review compiling evidence from multiple reviews into one accessible and usable document’ via ‘identification of component reviews, but no search for primary studies’. ‘Primary studies’ refer to original research studies or individual studies conducted by researchers to gather data first-hand.

Booth with colleagues later expanded the typology by introducing the concept of a review family construct and amalgamating various types of reviews for further refinement, such as traditional reviews, systematic reviews, review of reviews, rapid reviews, mixed-methods reviews, and purpose-specific reviews (for details, see Sutton et al., 2019 ).

2.1.2 Further development of the review typologies

Many classifications for review studies have been developed, and in the following section, we present more recent approaches. Paré et al. ( 2015 ), in another highly cited study (2,059 Google Scholar citations as of 20 April 2024) considered seven recurrent dimensions: the goal of the review, the scope of the review questions, the search strategy, the nature of the primary sources, the explicitness of the study selection, quality appraisal, and the methods used to analyse/synthesise the findings. Based on these dimensions, they formulated nine different literature review types: narrative reviews, descriptive reviews, scoping/mapping reviews, meta-analyses, qualitative systematic reviews, umbrella reviews, critical reviews, theoretical reviews, and realist reviews.

In Paré et al.’s ( 2015 ) classification, the review categories that differ from Grant and Booth’s ( 2009 ) classification are theoretical reviews, realist reviews, narrative reviews, and descriptive reviews, which we therefore describe them briefly. A theoretical review draws on conceptual and empirical studies to develop a conceptual framework or model using structured approaches, such as taxonomies, to discover patterns or commonalities. The aim of a realist review (also called a meta-narrative review) is to formulate explanations; such reviews ‘are theory-driven interpretative reviews which were developed to inform, enhance, extend, or alternatively supplement conventional systematic reviews by making sense of heterogeneous evidence about complex interventions applied in diverse contexts in a way that informs policy decision making’ (Paré et al., 2015 , p. 188). The purpose of a narrative review is to survey the existing literature on a particular subject or topic without necessarily seeking generalisations or cumulative insights from the material reviewed (Davies, 2000 ). Typically, such reviews do not detail the underpinning review processes or involve systematic and exhaustive searches of all pertinent literature. This category resembles Grant and Booth’s ( 2009 ) description of ‘literature reviews’ and overlaps with Samnani et al.’s ( 2017 ) narrative reviews, literature reviews, and overviews, resulting in a somewhat ambiguous typology. The aim of a descriptive review is to identify patterns and trends across a set of empirical studies within a specific research field, encompassing pre-existing propositions, theories, methodological approaches, or findings. To accomplish this objective, descriptive reviews collect, structure, and analyse numerical data that reflect the frequency distribution of research elements.

MacEntee ( 2019 ), Samnani et al. ( 2017 ), Schryen et al. ( 2020 ), and Taherdoost ( 2023 ) corroborated Grant and Booth’s ( 2009 ) and Paré et al.’s ( 2015 ) classifications, identifying various common review categories (see Table  1 ). In Samnani et al.’s ( 2017 ) classification, a distinct review type based on the previously mentioned categories is meta-synthesis , the aim of which is to provide explanations for phenomena, in contrast to meta-analysis, which focuses on quantitative outcomes.

Later, Schryen and Sperling ( 2023 ) introduced a slightly revised typology of literature review studies, which they applied to a meta-review of operations research. Their study distinguished nine types of literature reviews, newly introduced categories included tutorial reviews, selective reviews, algorithmic reviews, computational reviews, and meta-reviews. The objective of a tutorial review is to offer a research-oriented summary of principles, mathematical fundamentals, and concepts, aiming to inspire and direct future research endeavours. The authors’ emphasis on foundational aspects has often provided a launching pad for research advances. A selective review typically has a limited scope because it is not based on a thorough search of all relevant literature. This type of review concentrates on specific segments of the literature, such as journals, time periods, methodologies, or issues, to delve deeper into specific questions and phenomena. An algorithmic review focuses on advances in algorithms and frameworks in the literature that address a spectrum of problems. It employs either selective or comprehensive search strategies, predominantly examining algorithm-related sources. A computational review investigates algorithms and/or parameterisations proposed in the literature, largely considering implementations and computational studies, measurement efficiency, effectiveness, and different forms of robustness. Finally, Schryen and Sperling ( 2023 ) defined a meta-review as an overview of systematic reviews or a systematic review of reviews and pointed out that a meta-review can also be called an umbrella review (which is the case by Grant and Booth), again confirming the fuzzy nature of the currently available typologies. According to Schryen and Sperling ( 2023 ), meta-reviews primarily aim to furnish descriptive overviews of literature reviews, serving as tertiary studies that integrate evidence from multiple (qualitative or quantitative) reviews into unified and user-friendly documents (Becker & Oxman, 2008 ; Paré et al., 2015 ). In contrast to the previously mentioned perspectives, Schryen and Sperling ( 2023 ) argued that meta-reviews are not limited to addressing specific research questions but can also address a wide range of enquiries.

Chigbu et al. ( 2023 , pp. 5–6) emphasised that there ‘is a continuum of literature types’ (p. 4) and distinguished twelve different types of literature reviews, six of which were not covered by the classifications provided by previously mentioned studies: integrated reviews, interpretative reviews, iterative reviews, semi-systematic reviews, and bibliometric reviews. According to their approach, an integrative review builds ‘new knowledge based on the existing body of literature following a rationalist perspective’, an interpretative review ‘interprets what other scholars have written to put into specific perspectives’, and an iterative review is an ‘algorithm-based approach performed to collate all studies in a specific field of research’. Moreover, a meta-synthesis review examines and analyses qualitative study findings and is often employed to clarify specific concepts. Additionally, a semi-systematic review analyses the data and findings of other studies to address specific research inquiries, using a partial systematic review methodology. Lastly, a bibliometric review systematically examines the literature on a specific subject or research discipline by quantitatively measuring indicators such as authors, citations, journals, countries, and years of publications.

As previously noted in this paper, this detailed description of review types is instrumental in facilitating our investigation of various review studies in the realm of mathematics education.

2.2 Advancements in guidelines and protocols for review studies

Various researchers have developed guidelines, protocols, and statements to assist authors in conducting, evaluating, and reporting their review studies. This academic endeavour has predominantly focused on enhancing the rigour and transparency of systematic reviews, meta-analyses, and, more recently, scoping reviews. For instance, the population, intervention, comparison, and outcomes (PICO) model, originally conceived to support evidence-based healthcare, serves as a cornerstone for establishing review criteria, crafting research questions and search strategies, and delineating the characteristics of included studies or meta-analyses (Richardson et al., 1995 ). In response to the observed deficiencies in reporting standards within meta-analyses, an international consortium introduced the Quality of Reporting of Meta-Analyses (QUOROM) statement in 1996, primarily to enhance the reporting quality of meta-analyses involving randomised controlled trials (Moher et al., 1999 ). Subsequently, Moher et al. ( 2009 ) updated these guidelines, which are now known as the PRISMA guidelines, and incorporated various conceptual and methodological advances in systematic reviews and meta-analyses. Additionally, Shea et al. ( 2007 ) introduced the Assessment of Multiple Systematic Reviews (AMSTAR) checklist to evaluate methodological quality and guide the conduct of systematic reviews, while Grant and Booth ( 2009 ) developed the search, appraisal, synthesis, and analysis (SALSA) framework to analyse and characterise review types. Most recently, Page et al. ( 2021 ) updated the PRISMA guidelines, providing updated reporting standards that reflect advances in methods for identifying, selecting, appraising, and synthesising studies, with the aim of promoting more transparent, complete, and accurate reporting of systematic reviews and meta-analyses. An extension of PRISMA guidelines for scoping reviews, known as PRISMA-ScR, aids readers in understanding relevant terminology, core concepts, and key items for reporting scoping reviews (Tricco et al., 2018 ). Despite the value of these efforts, further studies are warranted, particularly comprehensive guidelines for each type of review studies.

2.3 Literature reviews in mathematics education

The preceding section delineates various types of review studies, underscoring their key methodological attributes. Within the realm of mathematics education, akin to other disciplines, literature review studies, particularly systematic reviews, and meta-analyses, received considerable attention (Cevikbas et al., 2022 ; Cevikbas & Kaiser, 2023 ; Kaiser & Schukajlow, 2024 ). However, the understanding of the prevailing characteristics of review studies in mathematics education, including prevalent review types, trends, gaps, and avenues for future improvement, remains limited.

Meta-reviews can offer a promising avenue for pinpointing research gaps, evaluating evidence quality, and informing policy and intervention strategies and guiding evidence-based decision-making processes by synthesizing findings from multiple review studies (Schryen & Sperling, 2023 ). In addition to meta-reviews, the bibliometric analyses serve to ascertain the scope of prior research, discern contemporary review trends, identify literature gaps, and propose future research agendas (Chigbu et al., 2023 ). While meta-reviews provide a comprehensive assessment of the literature, bibliometric analyses aid in systematically screening literature on a specific subject, topic, or research discipline by quantitatively measuring various indicators such as authors, citations, journals, countries, and years of publication. These methodological approaches hold promise for instituting a systematic, transparent, and reproducible review process, thereby augmenting the overall quality of reviews in mathematics education. Bibliometric techniques serve as valuable tools in literature reviews, guiding researchers by pinpointing influential works and impartially mapping the research landscape prior to in-depth exploration (Zupic & Cater, 2015 ).

Despite their significance, meta-reviews and bibliometric analyses remain seldom within the domain of mathematics education, signifying a substantial gap in the literature. Our comprehensive literature review underscores an urgent need for meta-review studies encompassing literature review studies in the realm of mathematics education. Additionally, while no bibliometric analysis study specifically focusing on review studies in mathematics education was identified, several bibliometric studies in mathematics education on various topics were noted, such as mathematics anxiety (Radevic & Milovanovic, 2023 ), problem-solving (Suseelan et al., 2022 ), and teacher noticing (Wei et al., 2023 ).

Overall, there exists a compelling need for meta-reviews enriched by bibliometric analyses to explore the current state of literature review research in mathematics education, and the current study aims to address this gap in a timely manner.

3 Methodology

3.1 literature search and manuscript selection process.

In this study, following the latest PRISMA guidelines (Page et al., 2021 ), we aimed to conduct a systematic review of previous review studies in mathematics education. Specifically, we employed the meta-review (umbrella review) method supplemented by bibliometric analyses. We processed the manuscript selection under three stages: identification, screening, and included.

3.1.1 Identification

On 10 January 2024 (last access), we conducted an extensive literature search using the WoS electronic database, which includes publications in high-ranking peer-reviewed journals and is widely acknowledged as a primary source of review and bibliometric data that meet high quality standards (Korom, 2019 ). WoS facilitates effective literature searches, supports various information purposes, and aids research topic mapping, trend monitoring as well as scholarly activity analysis (Birkle et al., 2020 ).

To comprehensively identify potentially relevant review studies in mathematics education, we developed an inclusive search query targeting specific terms in the titles, abstracts, and keywords of papers. The query comprised terms that we extracted from the typologies of literature reviews described in Chap. 2, particularly the more general, commonly used types of reviews:

( TOPIC ) ((literature review*OR literature survey* OR systematic review* OR rapid review* OR scoping review* OR critical review* OR meta-analysis OR narrative review* OR umbrella review* OR meta review* OR meta-review OR bibliometric review OR bibliometric analysis OR mapping review OR mixed-methods review OR integrative review OR interpretative review OR iterative review OR meta-synthesis OR descriptive review OR theoretical review OR realist review OR selective review OR algorithmic review OR computational review)) AND ( TOPIC ) ((math* OR geometry OR algebra OR calculus OR probability OR statistics OR arithmetic).

Based on these search strings, we conducted an online search that initially yielded 63,462 records.

3.1.2 Screening

In this stage, we applied data cleaning filters based on the manuscript inclusion and exclusion criteria (see Table  2 ). First, we electronically filtered the identified records based on language, resulting in the retention of 61,787 papers published in English. Subsequently, we narrowed down the selection to 10,098 papers using the following five categories of research areas within the WoS: ‘education/educational research, psychology, social sciences other topics, mathematics, or science technology other topics’. Following this categorisation, we further refined the dataset by excluding non-review papers and accessing 3,344 records within the ‘review article’ and ‘early access’ categories of the WoS database. We categorised records lacking a final publication date that had undergone peer review and acceptance as ‘early access’. Notably, to comprehensively capture publication trends, we imposed no restrictions on the publication years of the studies. In the subsequent phase, a meticulous manual screening of the titles, abstracts, and keywords of 3,344 papers led to the identification of 357 studies in mathematics education.

3.1.3 Included

Ultimately, after an extensive review of the full-text versions of initially identified 357 papers, 259 eligible review articles remained for analysis as these papers fulfilled our criteria comprehensively (see the Appendix for the list of included studies; see Fig.  1 for the flow diagram of the entire manuscript selection process). Subsequently, as detailed below, the data analysis process commenced with the inclusion of these eligible review papers in mathematics education.

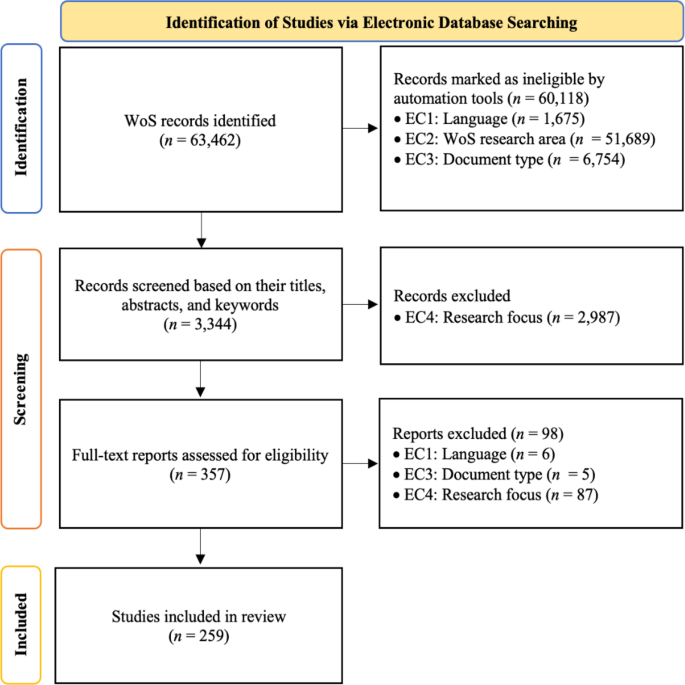
Flow diagram of the manuscript selection process

3.2 Data analysis

After incorporating 259 studies into this meta-review and bibliometric analysis, we compiled the identified records into a marked list on WoS. Subsequently, we exported the records into Excel, EndNote, and plain text file formats for analysis. The analysis consisted of content analysis and bibliometric analysis (see Fig.  2 , adapted from Wei et al., 2023 ).

For the content analysis, we meticulously organised the records using EndNote reference management software and Excel worksheets. We scrutinised the full-text versions of all included articles, coding them based on (1) publication year, (2) publisher, (3) review type, (4) number of included studies (sample size), (5) guidelines and protocols for the article selection process, and (6) the theoretical and conceptual framework of the studies.

Our coding manual, informed by prior studies (Cevikbas et al., 2022 , 2024 ), guided this process (see appendix for a sample of the coding manual). After completing the content analysis coding procedure, 20% of the papers ( n  = 52) were double-coded based on the initial coding protocol. The intercoder reliability, gauged at 0.92, signifies the presence of a coding system that exhibits satisfactory reliability (Creswell, 2013 ). Any discrepancies were addressed through discussions among the coders until consensus was reached.

For the bibliometric analysis, we employed VOSviewer software (version 1.6.20), which is widely recognised and extensively used in various fields, including the educational sciences (van Eck & Waltman, 2010 ). Chigbu et al. ( 2023 ) pointed out that the WoS database plays a pivotal role in facilitating bibliometric analyses across various disciplines. These analyses help establish trends in the development and application of knowledge within specific subjects and disciplines.

In our study, the bibliometric network presented in the results chapter consists of nodes and edges, with nodes representing entities such as publications, journals, researchers, or keywords. Edges denote relationships between pairs of nodes, indicating not only the presence or absence of connections but also conveying the intensity or strength of relationships (van Eck & Waltman, 2010 ). For distance-based approaches, the positioning of nodes in a bibliometric network reflects their approximate relatedness based on proximity.

Utilising VOSviewer software, we conducted (1) co-authorship analysis (authors and countries) to elucidate collaboration patterns and contributions, (2) co-occurrence analysis (focusing Author Keywords) to scrutinise knowledge structures and the distribution and development of key research topics in mathematics education, and (3) citation analysis to delve deeper into research influences and citation networks, drawing insights from the documents and sources.

This multifaceted approach allowed us to gain a comprehensive understanding of the bibliometric landscape and unravel collaborative structures, thematic foci, and the influence of key works on mathematics education.

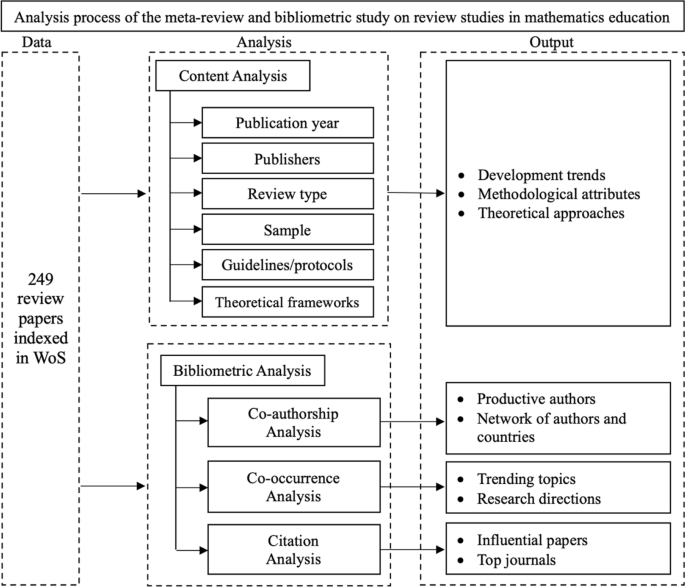
Analytical process for this study

In this chapter, we present the key results of the meta-review and bibliometric analyses divided into two main categories: an overview of the review studies in mathematics education based on the content analysis, addressing RQ1, and the results of the bibliometric analysis, addressing RQ2 – RQ5.

4.1 Overview of review studies in mathematics education (RQ1)

To discern the research trends and essential attributes of review studies in mathematics education, we conducted a content analysis within our meta-review to examine the 259 included review studies. Our analysis encompassed publication years, publishers, review types, guidelines, protocols used, sample sizes, and the theoretical and conceptual frameworks employed in these review studies. A general overview of the included studies is presented in Table  3 .

Our literature search with no restriction on the publication years yielded review studies published between 1996 and 2023, with a notable increase within the last five years (2019–2023, see Fig.  3 ).

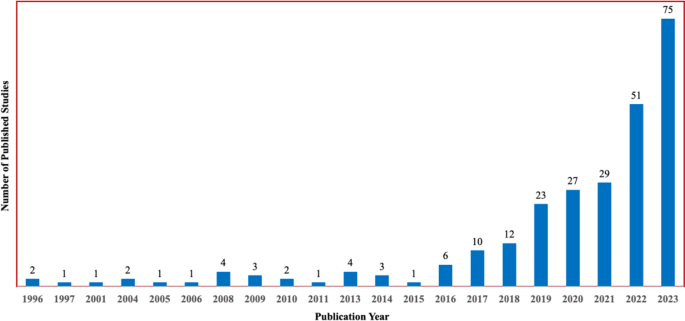
Distribution of publications from 1996 to 2023

The analysis showed that the Springer Group is the primary publisher of review articles in mathematics education, followed by Taylor & Francis, Elsevier, Sage, Frontiers, Wiley, MDPI, and the American Psychological Association (APA) (see Table  4 ). Other publishers published the remaining review articles ( n  = 43). This result may be attributed to the predominance of mathematics education journals published by Springer within the WoS database.

To explore the prevailing types of review studies in mathematics education, we scrutinised the review methodologies of the included studies, considering the review types presented earlier in Table  1 . The findings revealed that researchers conducted (according to their own classification) 10 different types of reviews in mathematics education as outlined in Fig.  4 .

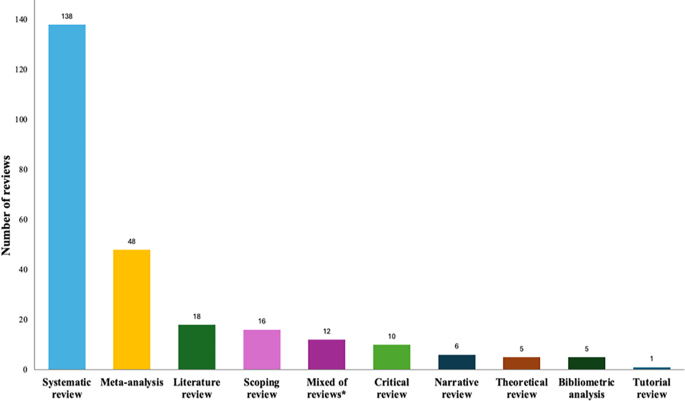
Types of review studies Note: *systematic reviews and meta-analyses ( n  = 6), systematic reviews and bibliometric analyses ( n  = 3), meta-analyses and narrative reviews ( n  = 2), and meta-analysis and critical review ( n  = 1)

Our analysis did not yield further review types in mathematics education. Time-related analysis showed that recent studies were systematic reviews, meta-analyses, literature reviews, and scoping reviews, whereas early examples of review studies in mathematics education were primarily narrative or critical reviews or were not explicitly classified according to review type by their authors. Figure  4 shows that some researchers ( n  = 18) described their studies as literature reviews using Grant and Booth’s ( 2009 ) generic term, without providing further details about the type of review.

To comprehend the methodologies employed by researchers to conduct reviews and select eligible studies, we conducted an analysis of the guidelines and protocols the researchers used. The findings revealed that the PRISMA guidelines were the most frequently employed ( n  = 121), aligning with the distribution of review types—PRISMA guidelines are basically recommended for systematic reviews and meta-analyses (Page et al., 2021 ). For scoping reviews, the guidelines developed by Arksey and O’Malley ( 2005 ) were the most prevalent and were used in seven studies. In six instances, researchers applied various guidelines (e.g. PICO or SALSA guidelines) sourced from the literature. Almost half of the studies ( n  = 125) did not specify the use of guidelines for conducting literature searches and selecting eligible studies. Additionally, three studies aimed to provide protocols for conducting review studies. Furthermore, seven studies were preregistered as review studies, following the Open Science Framework (OSF) and/or the International Prospective Register of Systematic Reviews (PROSPERO) protocol.

A prevalent discourse among researchers in review studies revolved around determining the most suitable number of studies to include in reviews. Our results revealed that the sample sizes of the included studies (i.e. the number of primary studies) in the field of mathematics education ranged from 8 to 3,485. Unfortunately, this information was not reported in 19 review articles. In the remaining 240 review articles, the average was 99 included studies, with an overall total of 23,761. Most of the studies ( n  = 202) had sample sizes of less than 100, with an average of 34 (see Table  5 ). Although we harboured concerns that the review studies identified in this investigation might not have been aptly named and conceptualised by their authors, we deliberately refrained from addressing this issue because it fell outside the scope of our study. While including a substantial number of studies is common and potentially suitable for bibliometric analyses and meta-analyses, conducting a systematic review, scoping review, or narrative review that critically analyses exceptionally high volumes of studies may pose challenges. In this meta-review, for example, we observed that five articles included more than 1,000 studies in the review process. Two studies, enriched by bibliometric analysis, took this approach, while another study was identified by the authors as a scoping review with a sample size of 2,433. Additionally, two studies were labelled as systematic reviews with sample sizes of 1,968, and 3,485, respectively.

Finally, we conducted a content analysis to scrutinise the theoretical and conceptual frameworks underpinning the included review studies in mathematics education. The findings revealed that out of 259 review studies, only 61 incorporated any theoretical or conceptual framework. Notably, a subset of studies ( n  = 14) was based on technology-related conceptual frameworks, such as Technological Pedagogical Content Knowledge (TPACK), frameworks pertaining to augmented and virtual reality, embodied design, artificial intelligence, big data, and the European Framework for the Digital Competence for Educators (DigCompEdu). Another prevalent category ( n  = 10) relied on frameworks related to the knowledge and competence of individuals (e.g. teachers and/or students), encompassing models such as the competence as continuum framework, TPACK, the didactic-mathematical knowledge and competencies model, mathematical content knowledge, pedagogical content knowledge, mathematical knowledge for teaching, teacher noticing competence, and an integrative model for the study of developmental competencies in minority children. Bronfenbrenner’s ecological theories (e.g. ecological theory of human development, bioecological model of human development, ecological systems theory, and ecological dynamics—a blend of dynamic-systems theory and ecological psychology) were employed by researchers in five review studies in mathematics education. In a limited subset of the studies, social and cultural theories (e.g. sociocultural theory, social learning theory, and cultural activity theory ( n  = 3)), cognitive theories (e.g. cognitive developmental theory ( n  = 2)), affective theories (e.g. self-determination theory and expectancy-value theory ( n  = 2)), linguistic theories ( n  = 2), and constructivist theories ( n  = 2) were used as frameworks. Additionally, researchers used conceptual frameworks concerning computational thinking ( n  = 2) and engagement ( n  = 3) alongside a few less frequently reported frameworks.

4.2 Results of the bibliometric analysis (RQ2–RQ5)

To identify productive and most cited authors, important journals, and countries of origin of the authors, along with the underlying research collaborations between researchers and countries, as well as research trends and key topics of review studies in mathematics education, we conducted a bibliometric analysis based on co-authorship, co-occurrence, and citations.

4.2.1 Co-authorship analysis

We conducted a co-authorship analysis according to authors and countries within the units of analysis.

Co-authorship and author analysis

The bibliometric analysis, using VOSviewer, revealed that 761 authors contributed to mathematics education, each of whom conducted at least one review study. The review papers were predominantly authored through collaboration, with most being written by two authors (30,2%), followed by three authors (20,2%), four authors (19,4%), a single author (10,1%), five authors (8,9%), six authors (6,2%), seven authors (3,5%), eight authors (1,6%), and nine authors (0,4%). These results showed that researchers primarily collaborate with their colleagues in conducting review studies—a practice vital for reducing workload and enhancing the quality of analyses—with the advantage of incorporating the various perspectives of different authors.

Table  6 highlights the top 17 authors who published a minimum of three review papers each. Notably, Lieven Verschaffel is the only scholar present in both lists of prolific and highly cited authors. The researchers listed in Table  7 , except Lieven Verschaffel, contributed to the field with a single review study. Consequently, while these researchers rank among most cited authors, the low total link strength (TLS) values indicate their limited collaboration with other scholars. The TLS was automatically calculated by VOSviewer and represents the overall intensity of co-authorship connections between a particular researcher and others. According to the co-authorship analysis, it is also noteworthy that many of the highly cited authors’ review studies typically date back over ten years, which is expected as citations tend to accumulate gradually over time. The results from the detailed citation analyses provided in Sect. 4.2.3.

Upon examining the research domains of prolific and highly cited authors, we found a diverse range of topics spanning mathematics education, psychology, educational psychology, special education, and neuroscience. This diversity highlights the interdisciplinary nature of research in mathematics education, with contributions to the literature review studies from psychologists and special education and neuroscience scholars alongside mathematics educators.

Figure  5 shows a co-authorship network map for the authors of the included review studies based on the TLS. We set the minimum number of documents for an author as one, which encompassed 761 authors who contributed to review papers in mathematics education. This bibliometric co-authorship analysis yielded 51 clusters, each containing a minimum of five items (researchers). The prominent co-authorship clusters included a green cluster (led by Lieven Verschaffel), a blue cluster (led by Gabriele Kaiser and Mustafa Cevikbas), a red cluster (led by Nelson Gena), and a yellow cluster (led by Diane P. Bryant). Nelson Gena had the highest number of collaboration links, with a TLS of 26, followed by Lieven Verschaffel (TLS = 22), Gabriele Kaiser (TLS = 16), Soyoung Park (TLS = 16), Tassia Bradford (TLS = 13), Diane P. Bryant (TLS = 12), Johannes König (TLS = 12), Mikyung Shin (TLS = 12), Min Wook Ok (TLS = 12), Bert de Smedt (TLS = 10), Fred Spooner (TLS = 10), Jihyun Lee (TLS = 10), Mustafa Cevikbas (TLS = 10), Rosella Santagata (TLS = 10), Sarah R. Powell (TLS = 10), and Thorsten Scheiner (TLS = 10).

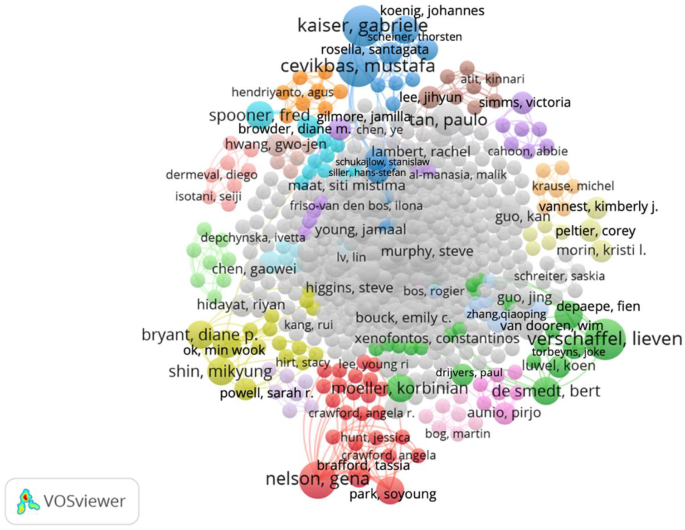
Co-authorship and author networks

Co-authorship and country analysis

We conducted a co-authorship–country analysis, setting the minimum number of documents for a country as one, and identified 50 countries. This selection resulted in five clusters, each containing a minimum of five items (countries).

The most prominent cluster was the green cluster, encompassing eight countries from various global regions: the United States (US; TLS = 30), Germany (TLS = 23), Australia (TLS = 21), China (TLS = 11), South Korea (TLS = 6), Sweden (TLS = 4), New Zealand (TLS = 2), and Jordan (TLS = 1). The US dominated research collaborations both within this cluster and overall.

The red cluster included nine countries, predominantly Nordic and European countries: Norway (TLS = 13), Finland (TLS = 7), Belgium (TLS = 6), the Netherlands (TLS = 6), Lithuania (TLS = 1), Portugal (TLS = 1), Luxembourg (TLS = 1), Scotland (TLS = 1), and Israel (TLS = 1).

The yellow cluster contained seven countries: Canada (TLS = 7), Malaysia (TLS = 7), Denmark (TLS = 3), Libya (TLS = 2), Singapore (TLS = 2), Indonesia (TLS = 1), and the United Arab Emirates (TLS = 1).

The blue cluster primarily highlighted European collaborations and included seven countries: England (TLS = 22), Switzerland (TLS = 4), Italy (TLS = 3), France (TLS = 3), Greece (TLS = 1), Chile (TLS = 1), and Saudi Arabia (TLS = 1).

Lastly, the purple cluster represented a network of predominantly South and North American countries featuring, among others, Brazil (TLS = 6), Ireland (TLS = 5), Mexico (TLS = 4), Ecuador (TLS = 2), and Cuba (TLS = 2)(See Fig. 6 ).

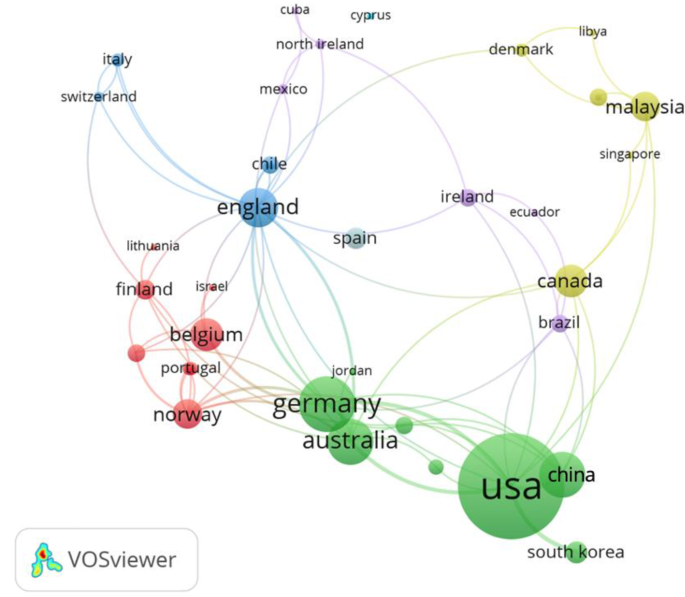
Co-authorship and country networks

4.2.2 Co-occurrence analysis

To explore the research hotspots within mathematics education, we ran a keyword co-occurrence analysis using Author Keywords.

Co-occurrence analysis based on author keywords

The author keyword co-occurrence analysis indicated that our repository contained 691 keywords (see Fig.  7 , left side), of which 23 met the minimum occurrence threshold of five occurrences ( n  = 5) (see Fig.  7 , right side). In the figure, the size of a node corresponds to the frequency of a keyword co-selected in review studies in mathematics education. The distance between any two keywords reflects their relative strength and topic similarity. Nodes within the same colour cluster indicate similar topics among these publications.

The red cluster comprises 11 closely related items, including ‘mathematics, meta-analysis, mathematics achievement, intervention, scoping review, bibliometric analysis, review, technology, learning disabilities, children, and math anxiety’. The green cluster emerges as the second prominent cluster, featuring 8 interrelated items such as ‘mathematics education, systematic review, systematic literature review, literature review, teacher education, education, teaching, and flipped classroom’. Lastly, the blue cluster consists of 4 items, namely ‘math, science, early childhood, and identity’.

Co-occurrence analysis of author keywords

Notably, the most frequently cited author keyword was ‘mathematics education’ ( n  = 55), followed by ‘systematic review’ ( n  = 44), ‘mathematics’ ( n  = 41), ‘meta-analysis’ ( n  = 34), ‘systematic literature review’ ( n  = 14), ‘literature review’ ( n  = 11), ‘teacher education’ ( n  = 9), ‘mathematics achievement’ ( n  = 8), ‘intervention’ ( n  = 6), ‘education’ ( n  = 6), ‘teaching’ ( n  = 6), ‘science’ ( n  = 6), ‘scoping review’ ( n  = 5), ‘bibliometric analysis’ ( n  = 5), ‘review’ ( n  = 5), ‘math’ ( n  = 5), ‘technology’ ( n  = 5), ‘flipped classroom’ ( n  = 5), ‘early childhood’ ( n  = 5), ‘children’ ( n  = 5), ‘identity’ ( n  = 5), ‘learning disabilities’ ( n  = 5), and ‘math anxiety’ ( n  = 6).

The keywords chosen by the authors highlighted the focus areas of reviews in mathematics education, emphasising themes such as mathematics achievement, teacher education, interventions, technology, and technology-enhanced approaches (e.g. flipped classrooms), special education, and early childhood education. Furthermore, the author keywords reflected the prevalent review types in mathematics education, specifically systematic reviews and meta-analyses. Additionally, they highlighted the interdisciplinary nature of reviews in mathematics education, encompassing both mathematics education and science education.

Furthermore, we conducted distinct author keyword co-occurrence analyses for review studies published within the periods of 2019 to 2023 and those preceding 2019, aiming to discern temporal trends in author keywords, particularly in recent years. The analysis yielded 606 keywords for the 2019–2023 period and 144 keywords for the period before 2019 (see Table  8 for the most popular 15 author keywords). A noteworthy disparity in prevalent keywords was observed between the two temporal segments. While predominant keyword regarding the review types prior to 2019 was meta-analysis, followed by literature review and systematic review, over the past five years, additional keywords such as scoping review and bibliometric analysis emerged, signalling an augmentation in the diversity of review types and methodologies. The findings indicated a notable increase in the popularity of systematic reviews over the past five years.

4.2.3 Citation analysis

To explore the most cited publications and journals in mathematics education, we conducted a citation analysis based on the units of analysis in documents and sources.

Citation and document analysis

The analysis of the 259 review papers in mathematics education included in this study indicated that they received a total of 7,050 citations between 1996 and 2023, averaging 251.79 citations per year and 27.22 citations per paper. Notably, 67% of these citations were received in the last five years (2019–2023).

The threshold for the minimum number of citations of documents was set at one, which 221 review studies out of 259 met. Figure  8 visualises the network between these review papers with the largest citation links and Table  9 shows the most cited documents. Not all the studies listed in Table  9 are among the top 10 studies with the highest TLS. Among them, only Gersten et al. ( 2009 ), Cheung and Slavin ( 2008 ), and Slavin and Lake ( 2008 ) are within the top 10 review studies in mathematics education with the highest TLS. While highly cited documents are influential in terms of direct references, the TLS metric provides additional insights into the collaborative relationships and connections between researchers and their work, which may not always correlate perfectly with citation counts as seen in our findings.

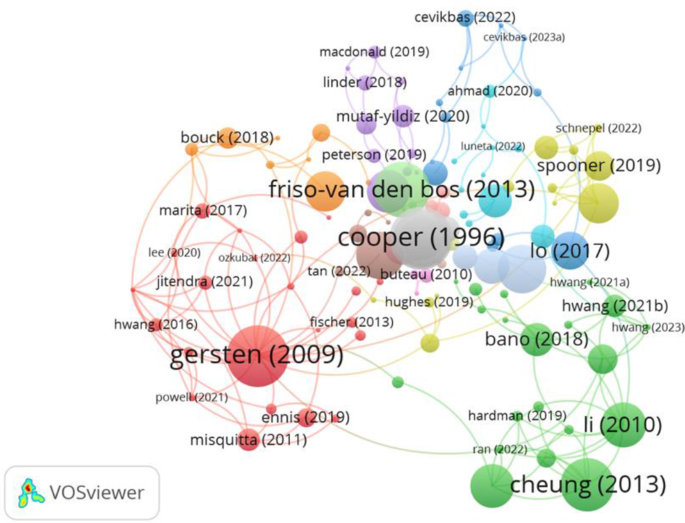
Our results showed that the largest number of citation links were for meta-analyses and systematic review studies. The most prominent review type among the most cited studies listed in Table  9 is meta-analysis ( n  = 6), followed by literature review ( n  = 2), systematic review ( n  = 1), and narrative review ( n  = 1). This result indicates the potential of meta-analysis studies in terms of citation performance. Most of these review studies were primarily published in high-ranking educational review journals ( n  = 6). Other review papers published in teacher education ( n  = 2), psychology ( n  = 1), and behavioural science and neuroscience journals ( n  = 1). These ten most cited review articles were all published in SSCI journals over a decade ago. Regarding research topics in the most cited papers, the dominant topics were mathematics achievement, content knowledge, working memory, learning disabilities, and educational technologies.

Specifically, we analysed the citation trends of the most cited 10 review papers over time and separately for the first five years after publication and the past five years (2019–2023). The results indicate a significant increase in the citations review studies have received in the last five years. We found that eight out of the ten most cited papers received more citations in the past five years (2019–2023) than in the first five years after their publication. The analysis revealed that the average annual citations for each paper ranged from 7 to 30. While the majority of these review studies ( n  = 8) received the least citations in the year of their publication, they received the most citations on average approximately 12 years after publication. This indicates that the peak citation period for review articles in mathematics education extends beyond the first decade following their publication.

Additionally, we investigated the ‘Enriched Cited References’ feature, which provides insight into why an author cited a particular reference; this beta enhancement is only available in selected journals (Clarivate, 2024 ). These references are presented to aid readers in quickly assessing sections of a review paper, allowing them to identify the most closely related or impactful references and infer their purpose. Articles containing enriched cited references are marked with the following labels (Clarivate, 2024 ):

Previously published research that contextualizes the current study within an academic domain.

References that supply the datasets, methodologies, concepts, and ideas directly utilized by the author or upon which the author’s work relies.

References introduced because the current study engages in a more thorough discussion.

References cited by the current study as yielding similar results. This may encompass methodological similarities or, in certain instances, replication of findings.

References noted by the current study as presenting contrasting results. This may also involve disparities in methodology or sample differences, influencing the outcomes.

The results, displayed in Table  10 , pertain to the classification of references based on the Enriched Cited References analysis conducted automatically by WoS. These results suggest that the most cited review studies in mathematics education were predominantly utilized by researchers to establish the background for their own research. Furthermore, these reviews also frequently employed to shape the discussion within the papers. In addition, some researchers utilize the mentioned most cited review studies to establish a conceptual, theoretical, or methodological basis. While the limited number of the studies cited these reviews to support their findings, they were not used to present opposing evidence. This suggests a reliance on existing literature review studies to inform, validate, or potentially challenge new research within the field.

Citation and source analysis

We conducted a citation source analysis and present the citation network map for the journals in Fig.  9 , listing the top 15 journals in Table  11 based on the citation and TLS metrics to represent the frequency of citations between articles in any two journals. The threshold for the minimum number of documents citing a source was one, and 103 records met the minimum number of citations of a source, also set at one. The network map shown in Fig.  9 indicates prominent clusters. The red cluster included 23 items (mostly special education, educational psychology, and educational review journals). The blue cluster included 16 items (predominantly educational psychology, educational technology, and educational review journals). The green cluster comprised 17 items (including mathematics and mathematics education journals, educational technology journals, and educational psychology journals).

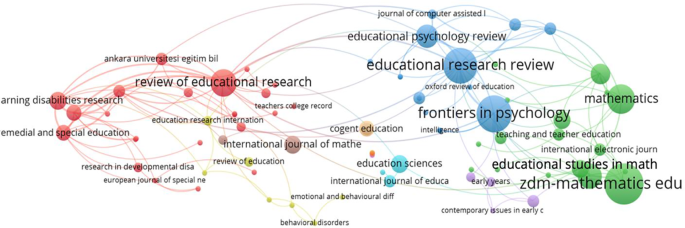
The number of articles and the distribution of journals across various research fields were as follows: 25 educational sciences journals (43 papers), 20 psychology and educational psychology journals (41 papers), 15 special education journals (32 papers), 12 mathematics education journals (52 papers), 10 educational review journals (41 papers), 9 educational technology journals (28 papers), 3 mathematics journals (14 papers), and 9 other journals (8 articles).

Our findings indicate that ZDM– Mathematics Education ( n  = 16) has, so far, published the most review studies focusing on mathematics education, which is not unexpected due to the origin of the journal as a review journal publishing only special issues, for which a review article is compulsory in each issue. This was followed by Frontiers in Psychology ( n  = 14), Educational Research Review ( n  = 13), and Mathematics ( n  = 10) (see Table  11 for the top 15 journals).

The results highlighted that the most frequently cited papers were often published in specific educational review journals (e.g. Review of Educational Research , Educational Research Review , and Educational Psychology Review ), psychology and educational psychology journals (e.g. Frontiers in Psychology , Educational Psychology Review , European Journal of Cognitive Psychology , and Psychological Bulletin ), special education journals (e.g. Exceptional Children , Learning Disabilities Research & Practice , Learning Disability Quarterly , and Remedial and Special Educati on), educational technology journals (e.g. Computers & Education , Journal of Computer Assisted Learning , and Education and Information Technologies ), and mathematics and mathematics education journals (e.g. ZDM– Mathematics Education , Educational Studies in Mathematics , and Mathematics ).

Although the most visible mathematics education journals in citation network map were ZDM– Mathematics Education and Educational Studies in Mathematics (see Fig.  9 ), as mentioned earlier, twelve mathematics education journals provided platforms for review studies. These were ZDM– Mathematics Education ( n  = 16), Educational Studies in Mathematics ( n  = 5), International Journal of Science and Mathematics Education ( n  = 5), International Journal of Mathematical Education in Science and Technology ( n  = 5), International Electronic Journal of Mathematics Education ( n  = 3), Mathematics Education Research Journal ( n  = 3), International Journal for Technology in Mathematics Education ( n  = 3), International Journal of Education in Mathematics, Science and Technology ( n  = 3), Journal for Research in Mathematics Education ( n  = 2), Canadian Journal of Science, Mathematics and Technology Education ( n  = 1), Journal für Mathematik-Didaktik ( n  = 1), and Research in Mathematics Education ( n  = 1).

5 Discussion, conclusions, and limitations

In this study, we conducted a meta-review of literature review studies in mathematics education, enriched by a comprehensive bibliometric analysis. This paper significantly contributes to scholarly discourse by unravelling nuanced research trends, the most common review methodologies, and prevalent theoretical approaches in review studies in mathematics education. Based on content and bibliometric analysis, it delves into the research foci, providing an understanding of the relevant academic landscape. Additionally, it illuminates intricate connections among researchers, countries, and journals, elucidating collaborative networks in mathematics education research.

5.1 Insights from the meta-review and implications

The findings revealed a significant increase in the number of literature reviews in mathematics education, particularly in the past five years; 79% of the reviews we examined were published during this period. Multiple factors may have contributed to this surge, including researchers’ increased publication output during the pandemic (Cevikbas & Kaiser, 2023 ; Nane et al., 2023 ), challenges in collecting empirical data during the pandemic crisis (Uleanya & Yu, 2023 ), the relatively high citation rates associated with literature review studies, the growing prestige of educational review journals based on their increased impact factors (Miranda & Garcia-Carpintero, 2018 ), and the publication of review-oriented special issues in mathematics education journals.

Our findings revealed a prevalence of systematic reviews and meta-analyses; however, researchers also conducted diverse types of reviews, including scoping reviews, critical reviews, narrative reviews, theoretical reviews, and tutorial reviews. This methodological diversity is important as the advantages of one method can potentially overcome the disadvantages of another and combining different approaches can mitigate disadvantages (Taherdoost, 2023 ). Furthermore, our study revealed that rapid reviews, meta-reviews, umbrella reviews, mapping reviews, mixed-methods reviews, integrative reviews, interpretative reviews, iterative reviews, meta-syntheses, descriptive reviews, realist reviews, selective reviews, algorithmic reviews, and computational reviews indexed in WoS were not represented in mathematics education. The well-established PRISMA guidelines offer a defined framework for systematic reviews and meta-analyses to assist researchers in conducting reviews while adhering to quality and transparency criteria (Moher et al., 2009 ; Page et al., 2021 ). This adherence may have encouraged researchers to undertake such reviews, and future advancements in the development of specific guidelines and methodologies for each review type may further motivate researchers to conduct other types of reviews in mathematics education more frequently.

There were nuanced overlaps between the review types, leading to ambiguous distinctions. For instance, the structural similarity between systematic reviews and scoping reviews has led to misunderstandings. Munn et al. ( 2018 ) confirm inconsistency and confusion regarding the differentiation between scoping reviews and systematic reviews and offered guidelines for this decision-making process: a systematic review is preferable when addressing specific questions regarding the feasibility, appropriateness, significance, or efficacy of a specific treatment or practice. However, if the authors intend to demarcate the research field and explore its potential size and scope, a scoping review is more appropriate. Grant and Booth ( 2009 ) and Munn et al. ( 2018 ) clarified that a scoping review is preparation for a systematic literature review, not a deep study for a systematic literature review. The diverse taxonomies proposed by researchers have contributed to this complexity, with some employing various terms for similar review characteristics, and others applying the same terms to studies with distinct review attributes. Consequently, a consensus regarding the categorisation of review studies, both in a broad context and specifically in mathematics education, remains elusive. We also observed instances of researchers labelling their reviews inaccurately. However, we refrained from judging the appropriateness of these terminologies as they fall outside the scope of our study and may be difficult to justify due to the ambiguity of the current typologies. Borges Migliavaca et al. ( 2020 ) expressed a similar concern, highlighting substantial disparities in review studies concerning their conceptualisation, conduct, reporting, risk of bias assessment, and data synthesis. They called for the evidence synthesis community to promptly develop guidance and reporting standards for review studies. Future researchers could potentially examine inconsistencies in the conducting of review studies and their categorisation in mathematics education. In this study, we distilled the various existing types of review studies to provide clear explanations of the main review types and to help researchers and readers understand the key characteristics of various review studies (see Chap. 2).

An additional noteworthy consideration pertains to the sample sizes of review studies. A prevalent discourse considers the appropriate number of studies to be included in a review, but establishing such a minimum or maximum number may be challenging and not appropriate because this depends on various contextual factors, such as the research area, topic, inclusion/exclusion criteria, and applied protocols. For example, in technical terms, a systematic review can be conducted with as few as two studies or as many as a thousand. A review study with a small sample (e.g. two or three studies) may be due to the literature search methods used or insufficient number of existing studies in a particular field, suggesting a limited demand for such a review. As previously noted, the primary function of review studies is to inform readers in the relevant field about published studies to address the challenge posed by an increasing number of studies and to identify trends and research gaps (Fusar-Poli & Radua, 2018 ). Conversely, although it is technically feasible to include a substantial number of studies in a review (e.g. 1,000 or 2,000), conducting a comprehensive analysis (e.g. content analysis) of such a large dataset can present major time, cost, storage, memory, bias, and security challenges (Cohen et al., 2015 ). Nevertheless, the findings of our study provide insight into this issue. Notably, the sample size of the studies we analysed varied from 8 to 3,485, with an average of 99. Notably, most of these studies (78%) had sample sizes of less than 100, with an average of 34. Although this observation does not serve as a prescriptive recommendation, it offers valuable insights into the typical sample sizes with which mathematics education researchers have tended to work in the past.

Furthermore, as evidenced by our findings, literature reviews may serve various purposes, such as assessing the use of theoretical models or conceptual and methodological approaches, or advancing new theories, concepts, or research models through critical appraisal of previous research within a specific subject area (Cooper, 1988 ). However, our findings also indicate that it is not common in practice to use or develop a theoretical or conceptual framework in mathematics education review studies. Only 24% of the reviewed studies explicitly reported employing a specific framework, and very few sought to formulate a framework based on the literature under scrutiny. The results highlighted the researchers’ interest in frameworks related to technology, knowledge, and competence models. A few studies incorporated grand theories, such as constructivism, sociocultural theory, and cognitive development theory.

It is remarkable that despite focusing on mathematics education, there is a notable scarcity of review studies employing content-specific frameworks in mathematics education, such as those centred on problem-solving, reasoning, and mathematical thinking. Only a minority of the studies used frameworks related to mathematical modelling and mathematical content knowledge. This observation may reflect a gap in the literature, suggesting a need for greater integration of domain-specific frameworks into review studies in mathematics education to enhance the depth and specificity of the studies. Moreover, this trend prompts a critical examination of potential underlying factors. One plausible explanation lies in the interdisciplinary nature of review studies in mathematics education, which draws contributions from diverse fields including psychology, educational technology, special education, and neuroscience. The diverse disciplinary backgrounds of the researchers may influence their preferences for frameworks that are not necessarily specific to mathematics education but rather draw from broader fields.

5.2 Insights from the bibliometric analyses and implications

The bibliometric analysis revealed contributions to mathematics education, with 761 authors from 50 countries conducting review studies. In future studies, researchers may consider conducting detailed analyses of how these initiatives have influenced the landscape of mathematics education, examining their specific impacts on various subfields, and assessing their overall influence.

Our findings reveal a notable participation in literature review studies within mathematics education by scholars from diverse backgrounds, including educational psychologists, mathematics educators, and specialists in special education and neuroscience. This multidisciplinary engagement underscores the broader interest of researchers beyond the field of mathematics education. Notably, co-authorship connections within US institutions were the most extensive. The leading countries that published review studies included the US, Germany, China, Australia, and England. A robust network emerged among researchers in North America, Europe, Asia, and Australia, emphasising collaboration opportunities that warrant exploration by African and South American researchers.

Systematic reviews and meta-analyses stood out as the predominant review types in mathematics education, both in terms of the number of publications and citation counts. Systematic reviews offer rigorous and comprehensive syntheses of existing literature on specific research questions, providing valuable insights, identifying gaps in knowledge, and informing evidence-based decision-making in various fields. Moreover, meta-analyses enhance statistical power, resolve conflicting findings, and offer more precise estimates of effect sizes by combining data from various sources. However, there is a discernible need to diversify the types of reviews conducted in mathematics education.

The findings underscore a significant surge in both the quantity of review studies and their citation counts within mathematics education especially over the recent five-year period (2019–2023). This trend suggests a prevalent practice among authors to draw upon previously published reviews to contextualize their own studies, frequently engaging in discussions and citing references to corroborate or challenge existing findings. Such reliance on established literature highlights the discipline’s emphasis on leveraging prior knowledge to inform and substantiate new research endeavours.

The most cited review papers were associated with specific educational review journals, educational psychology journals, special education journals, educational technology journals, and mathematics education journals, further highlighting the interdisciplinary nature of impactful research in the field. The results revealed that ZDM– Mathematics Education , Educational Studies in Mathematics , International Journal of Science and Mathematics Education , and International Journal of Mathematical Education in Science and Technology were the key mathematics education journals committed to publishing review studies. The performance of these journals, particularly in recent years, reflects the escalating significance of review studies in mathematics education. Nevertheless, the limited visibility of some mathematics education journals in publishing review studies could be attributed, among other factors, to their restricted representation in the WoS database or to the overall small number of studies published yearly in particular mathematics education journals.

Prominent research topics in mathematics education review studies are digital technologies, technology-enhanced approaches (e.g. flipped classrooms), teacher education, mathematics achievement, early childhood education, and learning disabilities. Recent technological advances, including artificial intelligence and augmented/virtual reality, may soon attract mathematics education researchers’ attention to emerging technologies (Cevikbas, Bulut et al., 2023 ; Cevikbas, Greefrath et al., 2023 ). In addition to technology-enhanced mathematics education and special education, researchers have also explored the cognitive and affective aspects of learning and teaching mathematics.

In short, the absence of high-quality research syntheses may impede theoretical and conceptual advances within mathematics education (Webster & Watson, 2002 ). Therefore, future researchers may endeavour to develop discipline-specific standards and guidelines for conducting various types of review studies in mathematics education. Moreover, they could focus on expanding the content of mathematics education journals to accommodate a greater number of review studies. The scientific influence of review journals may also provide an opportunity to establish a dedicated review journal with a pronounced focus on mathematics education.

5.3 Limitations and conclusion

Finally, we want to point out that in this comprehensive meta-review, enriched by bibliometric analysis, we meticulously compiled and scrutinised the largest dataset of reviews in mathematics education available within the WoS database. Although this was a substantial sample ( n  = 259) that was reasonably representative of published review studies in mathematics education, it is important to acknowledge certain limitations. Our search was confined to WoS, and we specifically focused on review articles published in English. It is worth noting that the characteristics of review studies published in journals, international handbooks, or conference proceedings not indexed in WoS or published in a language other than English could potentially differ from those we examined. In addition, despite studies indexed in WoS theoretically being of high quality, we identified inconsistencies and variability in the review studies we examined, and it is possible that a more extensive search would have yielded different results.

In conclusion, we advocate producing high-quality review papers that adeptly synthesise available knowledge to improve professional practice (Templier & Paré, 2015 ). Such efforts may further advance mathematics education and contribute to the continuous improvement of teaching and learning activities, despite the demanding nature of comprehensive review studies.

Arksey, H., & O’Malley, L. (2005). Scoping studies: Towards a methodological framework. International Journal of Social Research Methodology , 8 (1), 19–32.

Article   Google Scholar  

Becker, L. A., & Oxman, A. D. (2008). Overviews of reviews. In J. P. T. Higgins, & S. Green (Eds.), Cochrane handbook for systematic reviews of interventions (pp. 607–631). Wiley.

Birkle, C., Pendlebury, D. A., Schnell, J., & Adams, J. (2020). Web of Science as a data source for research on scientific and scholarly activity. Quantitative Science Studies , 1 (1), 363–376.

Boote, D., & Beile, N. (2005). Scholars before researchers: On the centrality of the dissertation literature review in research preparation. Educational Researcher , 34 (6), 3–15.

Borges Migliavaca, C., Stein, C., Colpani, V., et al. (2020). How are systematic reviews of prevalence conducted? A methodological study. BMC Medical Research Methodology , 20 , 96.

Cevikbas, M., & Kaiser, G. (2023). Can flipped classroom pedagogy offer promising perspectives for mathematics education on pandemic-related issues? A systematic literature review. ZDM–Mathematics Education , 55 , 177–191.

Cevikbas, M., Kaiser, G., & Schukajlow, S. (2022). A systematic literature review of the current discussion on mathematical modelling competencies: State-of-the-art developments in conceptualizing, measuring, and fostering. Educational Studies in Mathematics , 109 (2), 205–236.

Cevikbas, M., Greefrath, G., & Siller, H. S. (2023). Advantages and challenges of using digital technologies in mathematical modelling education–a descriptive systematic literature review. Frontiers in Education , 8 , 1142556.

Cevikbas, M., Bulut, N., & Kaiser, G. (2023). Exploring the benefits and drawbacks of AR and VR technologies for learners of mathematics: Recent developments. Systems , 11 (5), 244.

Cevikbas, M., König, J., & Rothland, M. (2024). Empirical research on teacher competence in mathematics lesson planning: Recent developments. ZDM–Mathematics Education , 56 , 101–113.

Cheung, A. C., & Slavin, R. E. (2013). The effectiveness of educational technology applications for enhancing mathematics achievement in K-12 classrooms: A meta-analysis. Educational Research Review , 9 , 88–113.

Chigbu, U. E., Atiku, S. O., & Du Plessis, C. C. (2023). The science of literature reviews: Searching, identifying, selecting, and synthesising. Publications , 11 (1), 2.

Clarivate (2024). Citation context in Web of Science . URL.

Cochrane, A. L. (1979). 1931–1971: A critical review with particular reference to the medical profession. In G. Teeling Smith, & N. Wells (Eds.), Medicines for the year 2000 (pp. 2–12). Office of Health Economics.

Cohen, B., Vawdrey, D. K., Liu, J., Caplan, D., Furuya, E. Y., Mis, F. W., & Larson, E. (2015). Challenges associated with using large data sets for quality assessment and research in clinical settings. Policy Politics & Nursing Practice , 16 (3–4), 117–124.

Cooper, H. M. (1988). Organizing knowledge syntheses: A taxonomy of literature reviews. Knowledge in Society , 1 (1), 104–126.

Google Scholar  

Cooper, H., Nye, B., Charlton, K., Lindsay, J., & Greathouse, S. (1996). The effects of summer vacation on achievement test scores: A narrative and meta-analytic review. Review of Educational Research , 66 (3), 227–268.

Creswell, J. W. (2013). Qualitative inquiry and research design; choosing among five approaches . Sage.

Davies, P. (2000). The relevance of systematic reviews to educational policy and practice. Oxford Review of Education , 26 (3–4), 365–378.

Depaepe, F., Verschaffel, L., & Kelchtermans, G. (2013). Pedagogical content knowledge: A systematic review of the way in which the concept has pervaded mathematics educational research. Teaching and Teacher Education , 34 , 12–25.

DeStefano, D., & LeFevre, J. A. (2004). The role of working memory in mental arithmetic. European Journal of Cognitive Psychology , 16 (3), 353–386.

Friso-van den Bos, I., Van der Ven, S. H., Kroesbergen, E. H., & Van Luit, J. E. (2013). Working memory and mathematics in primary school children: A meta-analysis. Educational Research Review , 10 , 29–44.

Fusar-Poli, P., & Radua, J. (2018). Ten simple rules for conducting umbrella reviews. Evidence-Based Mental Health , 21 (3), 95.

Geary, D. C. (1996). Sexual selection and sex differences in mathematical abilities. Behavioral and Brain Sciences , 19 (2), 229–247.

Geng, Y., Chen, W., Liu, Z., Chiu, A. S., Han, W., Liu, Z., & Cui, X. (2017). A bibliometric review: Energy consumption and greenhouse gas emissions in the residential sector. Journal of Cleaner Production , 159 , 301–316.

Gersten, R., Chard, D. J., Jayanthi, M., Baker, S. K., Morphy, P., & Flojo, J. (2009). Mathematics instruction for students with learning disabilities: A meta-analysis of instructional components. Review of Educational Research , 79 (3), 1202–1242.

Grant, M. J., & Booth, A. (2009). A typology of reviews: An analysis of 14 review types and associated methodologies. Health Information & Libraries Journal , 26 (2), 91–108.

Hart, C. (2018). Doing a literature review: Releasing the research imagination (2nd edition). Sage.

Kaiser, G., & Schukajlow, S. (2024). Literature reviews in mathematics education and their significance to the field. ZDM–Mathematics Education , 56 , 1–3.

Ketcham, C. M., & Crawford, J. M. (2007). The impact of review articles. Laboratory Investigation , 87 (12), 1174–1185.

Korom, P. (2019). A bibliometric visualization of the economics and sociology of wealth inequality: A world apart? Scientometrics , 118 , 849–868.

Kunisch, S., Denyer, D., Bartunek, J. M., Menz, M., & Cardinal, L. B. (2023). Review research as scientific inquiry. Organizational Research Methods , 26 (1), 3–45.

Li, Q., & Ma, X. (2010). A meta-analysis of the effects of computer technology on school students’ mathematics learning. Educational Psychology Review , 22 , 215–243.

MacEntee, M. I. (2019). A typology of systematic reviews for synthesising evidence on health care. Gerodontology , 36 (4), 303–312.

Martin, D. B. (2009). Researching race in mathematics education. Teachers College Record , 111 (2), 295–338.

Martínez, M. A., Cobo, M. J., Herrera, M., & Herrera-Viedma, E. (2015). Analyzing the scientific evolution of social work using science mapping. Research on Social Work Practice , 25 (2), 257–277.

Miranda, R., & Garcia-Carpintero, E. (2018). Overcitation and overrepresentation of review papers in the most cited papers. Journal of Informetrics , 12 (4), 1015–1030.

Moher, D., Cook, D. J., Eastwood, S., Olkin, I., Rennie, D., & Stroup, D. F. (1999). Improving the quality of reporting of meta-analysis of randomized controlled trials: The QUOROM statement. Lancet , 354 , 1896–1900.

Moher, D., Liberati, A., Tetzlaff, J., Altman, D. G., & the PRISMA Group. (2009). Preferred reporting items for systematic reviews and meta-analyses: The PRISMA statement. Plos Medicine , 6(7), e1000097.

Munn, Z., Peters, M. D., Stern, C., Tufanaru, C., McArthur, A., & Aromataris, E. (2018). Systematic review or scoping review? Guidance for authors when choosing between a systematic or scoping review approach. BMC Medical Research Methodology , 18 , 1–7.

Nane, G. F., Robinson-Garcia, N., van Schalkwyk, F., & Torres-Salinas, D. (2023). COVID-19 and the scientific publishing system: Growth, open access and scientific fields. Scientometrics , 128 (1), 345–362.

Page, M. J., McKenzie, J. E., Bossuyt, P. M., Boutron, I., Hoffmann, T. C., Mulrow, C. D., McDonald, S., & Moher, D. (2021). (2021). The PRISMA 2020 statement: an updated guideline for reporting systematic reviews. BMJ , 372 (71), 1–9.

Paré, G., Trudel, M. C., Jaana, M., & Kitsiou, S. (2015). Synthesizing information systems knowledge: A typology of literature reviews. Information & Management , 52 (2), 183–199.

Radevic, L., & Milovanovic, I. (2023). Current trends in math anxiety research: A bibliometric approach. International Journal of Science and Mathematics Education . https://doi.org/10.1007/s10763-023-10424-4 .

Richardson, W. S., Wilson, M. C., Nishikawa, J., & Hayward, R. S. (1995). The well-built clinical question: A key to evidence-based decisions. ACP Journal Club , 123 (3), A12–A13.

Samnani, S. S., Vaska, M., Ahmed, S., & Turin, T. C. (2017). Review typology: The basic types of reviews for synthesizing evidence for the purpose of knowledge translation. Journal of the College of Physicians and Surgeons Pakistan , 27 (10), 635–641.

Schryen, G., & Sperling, M. (2023). Literature reviews in operations research: A new taxonomy and a meta review. Computers & Operations Research , 106269.

Schryen, G., Wagner, G., Benlian, A., & Paré, G. (2020). A knowledge development perspective on literature reviews: Validation of a new typology in the IS field. Communications of the AIS , 46 , 134–186.

Shea, B. J., Grimshaw, J. M., Wells, G. A., Boers, M., Andersson, N., Hamel, C., & Bouter, L. M. (2007). Development of AMSTAR: A measurement tool to assess the methodological quality of systematic reviews. BMC Medical Research Methodology , 7 , 1–7.

Slavin, R. E., & Lake, C. (2008). Effective programs in elementary mathematics: A best-evidence synthesis. Review of Educational Research , 78 (3), 427–515.

Song, Y., Chen, X., Hao, T., Liu, Z., & Lan, Z. (2019). Exploring two decades of research on classroom dialogue by using bibliometric analysis. Computers & Education , 137 , 12–31.

Suseelan, M., Chew, C. M., & Chin, H. (2022). Research on Mathematics Problem solving in Elementary Education conducted from 1969 to 2021: A bibliometric review. IJEMST , 10 (4), 1003–1029.

Sutton, A., Clowes, M., Preston, L., & Booth, A. (2019). Meeting the review family: Exploring review types and associated information retrieval requirements. Health Information & Libraries Journal , 36 (3), 202–222.

Taherdoost, H. (2023). Towards nuts and bolts of conducting literature review: A typology of literature review. Electronics , 12 (4), 800.

Templier, M., & Paré, G. (2015). A framework for guiding and evaluating literature reviews. Communications of the Association for Information Systems , 37 (1), 6.

Tricco, A. C., Lillie, E., Zarin, W., O’Brien, K. K., Colquhoun, H., Levac, D., Moher, D., Peters, M. D., Horsley, T., Weeks, L., Hempel, S., et al. (2018). PRISMA extension for scoping reviews (PRISMA-ScR): Checklist and explanation. Annals of Internal Medicine , 169 (7), 467–473.

Uleanya, C., & Yu, K. (2023). Data collection in times of pandemic: A self-study and revisit of research practices during a crisis. Sage Open , 13 (1), 21582440231160698.

van Eck, N., & Waltman, L. (2010). Software survey: VOSviewer, a computer program for bibliometric mapping. Scientometrics , 84 (2), 523–538.

Webster, J., & Watson, R. T. (2002). Analyzing the past to prepare for the future: Writing a literature review. MIS Quarterly , 26 (2), xiii–xxiii.

Wei, Y., Zhang, Q., Guo, J., & Chen, M. (2023). Learning to teach through noticing: A bibliometric review of teacher noticing research in mathematics education during 2006–2021. Humanities and Social Sciences Communications , 10 (1), 1–15.

Zupic, I., & Cater, T. (2015). Bibliometric methods in management and organization. Organizational Research Methods , 18 (3), 429–472.

Download references

Open Access funding enabled and organized by Projekt DEAL.

Author information

Authors and affiliations.

Humboldt-Universität zu Berlin Institute of Educational Sciences, Unter den Linden 6, 10099, Berlin, Germany

Mustafa Cevikbas

Faculty of Education, Universität Hamburg, Von-Melle-Park 8, 20146, Hamburg, Germany

Gabriele Kaiser

Nord University, Bodø, Norway

Institute of Mathematics Education and Computer Science Education, Universität Münster, Henriette-Son Str. 19, 48149, Münster, Germany

Stanislaw Schukajlow

You can also search for this author in PubMed   Google Scholar

Corresponding author

Correspondence to Mustafa Cevikbas .

Additional information

Publisher’s note.

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

The original online version of this article was revised: the style of table 1 was not good

The electronic supplementary material includes the list of the reviewed studies and a sample of the coding manual.

Rights and permissions

Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/ .

Reprints and permissions

About this article

Cevikbas, M., Kaiser, G. & Schukajlow, S. Trends in mathematics education and insights from a meta-review and bibliometric analysis of review studies. ZDM Mathematics Education 56 , 165–188 (2024). https://doi.org/10.1007/s11858-024-01587-7

Download citation

Accepted : 02 May 2024

Published : 15 May 2024

Issue Date : May 2024

DOI : https://doi.org/10.1007/s11858-024-01587-7

Share this article

Anyone you share the following link with will be able to read this content:

Sorry, a shareable link is not currently available for this article.

Provided by the Springer Nature SharedIt content-sharing initiative

• Bibliometric analysis
• Critical review
• Literature review
• Mapping review
• Mathematics education
• Meta-review
• Narrative review
• Systematic review
• Scoping review
• Theoretical review
• Tutorial review
• Umbrella review
• Find a journal
• Publish with us
• Track your research