my homework lesson 2 estimate sums and differences

$enVision Math Answer Key$

Envision Math Grade 5 Answer Key Topic 2.3 Estimating Sums and Differences

Envision math 5th grade textbook answer key topic 2.3 estimating sums and differences.

$Envision Math Grade 5 Answers Topic 2.3 Estimating Sums and Differences 1$

Another Example How can you estimate differences? Estimate 22.8 – 13.9.

$Envision Math Grade 5 Answers Topic 2.3 Estimating Sums and Differences 3$

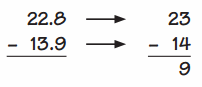
Question 1. Which estimate is closer to the actual difference? How can you tell without subtracting? Answer:

Question 2. When is it appropriate to estimate an answer? Answer:

Guided Practice*

Do you know HOW?

In 1 through 6, estimate the sums and differences.

Question 1. 49 + 22 Answer:

Question 2. 86 – 18 Answer:

Question 3. 179 + 277 Answer:

Question 4. 232 – 97 Answer:

Question 5. 23.8 – 4.7 Answer:

Question 6. 87.2 + 3.9 Answer:

Do you UNDERSTAND?

Question 7. Give an example of when estimating is useful. Answer:

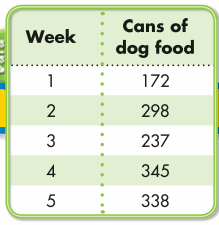
Question 8. The students in the example at the top collected more cans of dog food in week 4 than in week 3. Estimate about how many more. Answer:

$Envision Math Grade 5 Answers Topic 2.3 Estimating Sums and Differences 5$

Independent Practice

In 9 through 24, estimate each sum or difference

$Envision Math Grade 5 Answers Topic 2.3 Estimating Sums and Differences 11$

Question 21. 3,205 – 2,812 Answer;

Question 22. 93 – 46 Answer;

Question 23. 1,052 + 963 Answer:

Question 24. 149 – 51 Answer:

In 25 through 39, estimate each sum or difference.

$Envision Math Grade 5 Answers Topic 2.3 Estimating Sums and Differences 23$

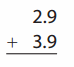
Question 33. 77.11 – 8.18 Answer:

Question 34. 35.4 – 7.8 Answer:

Question 36. 89.66 – 27.9 Answer:

Question 37. 22.8 + 49.2 + 1.7 Answer:

Question 38. 67.5 – 13.7 Answer:

Question 39. $9.10 + $48.50 + $5.99 Answer:

Problem Solving

Question 40. Writing to Explain The cost of one CD is $16.98, and the cost of another CD is $9.29. Brittany estimated the cost of these two CDs to be about $27. Did she overestimate or underestimate? Explain. Answer:

Question 41. Martha cycled 14 miles each day on Saturday and Monday, and 13 miles each day on Tuesday and Thursday. How many miles did she cycle in all? Answer:

Question 42. One fifth-grade class has 11 boys and 11 girls. A second fifth-grade class has 10 boys and 12 girls. There are 6 math teachers. To find the total number of fifth-grade students, what information is not needed? A. The number of girls in the first class. B. The number of boys in the first class. C. The number of math teachers. D. The number of boys in the second class. Answer:

Question 43. On vacation, Steven spent $13 each day on Monday and Tuesday. He spent $9 each day on Wednesday and Thursday. If Steven brought $56 to spend, how much did he have left to spend? Answer:

Question 44. Estimate 74.05 + 9.72 + 45.49 by rounding to the nearest whole number. What numbers did you add? A. 75, 10, and 46 B. 74.1, 9.7, and 45.5 C. 74, 10, and 45 D. 75, 10, and 50 Answer:

$Envision Math 5th Grade Answer Key Topic 2.3 Estimating Sums and Differences 31$

Number Patterns

$Envision Math 5th Grade Answer Key Topic 2.3 Estimating Sums and Differences 50$

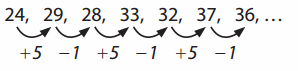
The following numbers form a pattern. 3, 7, 11, 15, 19, … In this case the pattern is a simple one. The pattern is add 4. Some patterns are more complicated. Look at the following pattern. 20, 24, 30, 34, 40, 44, 50, … In this case, the pattern is add 4, add 6. Look for a pattern. Find the next two numbers.

Question 1. 9, 18, 27, 36, 45, … Answer:

Question 2. 90, 80, 70, 60, 50, … Answer:

Question 3. 2, 102, 202, 302, … Answer:

Question 4. 26, 46, 66 , 86, … Answer:

Question 5. 20, 31, 42, 53, 64, … Answer:

Question 6. 100, 92, 84, 76, 68, … Answer:

Question 7. 1, 3, 9, 27, … Answer:

Question 8. 800, 400, 200, 100, … Answer:

Question 9. 20, 21, 19, 20, 18, 19, 17, … Answer:

Question 10. 10, 11, 21, 22, 32, 33, … Answer:

Question 11. 25, 32, 28, 35, 31, 38, … Answer:

Question 12. 5, 15, 10, 20, 15, 25, 20, … Answer:

Question 13. The following numbers are called Fibonacci numbers. 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, … Explain how you could find the next two numbers. Answer:

Question 14. Write a Problem Write a number pattern that involves two operations. Answer:

McGraw Hill My Math Grade 5 Chapter 9 Lesson 9 Answer Key Estimate Sums and Differences

All the solutions provided in McGraw Hill Math Grade 5 Answer Key PDF Chapter 9 Lesson 9 Estimate Sums and Differences will give you a clear idea of the concepts.

McGraw-Hill My Math Grade 5 Answer Key Chapter 9 Lesson 9 Estimate Sums and Differences

Math in My World

$McGraw Hill My Math Grade 5 Chapter 9 Lesson 9 Answer Key Estimate Sums and Differences 1$

Helpful Hint The ≈ means about or approximately equal to.

$McGraw Hill My Math Grade 5 Chapter 9 Lesson 9 Answer Key Estimate Sums and Differences_2$

Guided Practice

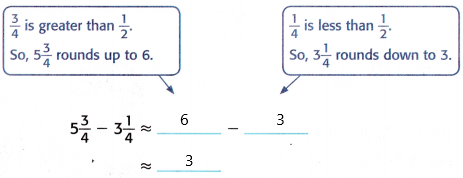
Estimate by rounding each mixed number to the nearest whole number.

Question 1. 2$\frac{1}{8}$ + 3$\frac{5}{8}$ Answer: The above-given: 2 1/8 + 3 5/8 1/8 is less than 1/2, so 1/8 rounds up to 0. 2 + 0 = 2 5/8 rounds up to 1. 3 + 1 = 4 Now add: 2 + 4 = 6 Therefore, 2$\frac{1}{8}$ + 3$\frac{5}{8}$ = 6

Talk Math Explain how you would estimate 8$\frac{4}{7}$ – 4$\frac{2}{7}$. Answer: The above-given: 8 4/7 – 4 2/7 Explanation: – Take the given fraction. – Convert the given fraction into decimals by dividing the numerator by the denominator. – If the obtained number to the right of the decimal point is 0.5 or more, then add 1 to the left of the decimal point and remove the decimal part. – If the obtained number to the right of the decimal point is lesser than 0.5, then leave the number to the left of the decimal point and remove the decimal part. – Write the new number as a rounded number. 4/7 = 0.6; so it can be rounded up to 1. 8 + 1 = 9 2/7 = 0.3 The number right of the decimal point is .3 which is lesser than .5. So, add 0 to the left of the decimal point and remove the decimal part. 4 + 0 = 4 Now subtract: 9 – 4 = 5 Therefore, 8$\frac{4}{7}$ – 4$\frac{2}{7}$ = 5.

Question 2. 5$\frac{2}{9}$ – 3$\frac{2}{3}$ Answer: The above-given: 5 2/9 – 3 2/3 – Take the given fraction. – Convert the given fraction into decimals by dividing the numerator by the denominator. – If the obtained number to the right of the decimal point is 0.5 or more, then add 1 to the left of the decimal point and remove the decimal part. – If the obtained number to the right of the decimal point is lesser than 0.5, then leave the number to the left of the decimal point and remove the decimal part. – Write the new number as a rounded number. The actual value of 2/9 = 0.2222 The round-off value is 0 because .2 is less than the .5 5 2/9 = 5 + 0 = 5 Now take another mixed fraction and convert it to the whole number. 3 2/3: The actual value of 2/3 is 0.66666 The round-off value is 1 because .6 is greater than .5 so we have to add 1 as per the above rules. 3 + 1 = 4 Now subtract 5 – 4 = 1 Therefore, 5$\frac{2}{9}$ – 3$\frac{2}{3}$ = 1.

Question 3. 3$\frac{3}{5}$ + 1$\frac{1}{5}$ Answer: The above-given: 3 3/5 + 1 1/5 Take the first fraction and round off: 3 3/5: 3/5 can be written as 1 because the actual value of the 3/5 is 0.6 .6 is greater than .5 so we have to add 1. 3 + 1 = 4 Take another fraction: 1 1/5: 1/5 can be written as 0 because the actual value of 1/5 is 0.2 .2 is less than .5 so leave the number to the left of the decimal point and remove the decimal part. 1 + 0 =1 Now add: 4 + 1 = 5 Therefore, 3$\frac{3}{5}$ + 1$\frac{1}{5}$ = 5

Independent Practice

$McGraw Hill My Math Grade 5 Chapter 9 Lesson 9 Answer Key Estimate Sums and Differences 4$

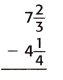
Question 7. 6$\frac{7}{10}$ – 1$\frac{1}{5}$ Answer: The above-given: 6 7/10 – 1 1/5 Take the first fraction and round off to the nearest whole number: 6 7/10: 7/10 can be rounded off to 1 because the actual value of 7/10 is 0.7 and moreover, .7 is greater than .5 so we have to add 1. 6 + 1 = 7 Now take another fraction: 1 1/5: 1/5 can be rounded to 0 because the actual value of 1/5 is 0.2 .2 is less than .5 so leave the number to the left of the decimal point and remove the decimal part. 1 + 0 = 1 Now subtract: 7 – 1 = 6 Therefore, 6$\frac{7}{10}$ – 1$\frac{1}{5}$ = 6.

Question 8. 8$\frac{11}{12}$ + 4$\frac{1}{3}$ Answer: The above-given: 8 11/12 + 4 1/3 Take the first fraction and round off to the nearest whole number: 8 11/12: 11/12 can round to 1 because the actual value of 11/12 is 0.9 .9 is greater than .5 so we have to add 1. 8 + 1 = 9 Take another fraction: 4 1/3: 1/3 can be rounded to 0 because its actual value is 0.3 .3 is less than .5 so leave the number to the left of the decimal point and remove the decimal part. 4 + 0 = 4 Now add: 9 + 4 = 13 Therefore, 8$\frac{11}{12}$ + 4$\frac{1}{3}$ = 13.

Question 9. 15$\frac{3}{7}$ – 3$\frac{4}{7}$ Answer: The above-given: 15 3/7 – 3 4/7 Take the first fraction and round off to the nearest whole number: 15 3/7: 3/7 can be rounded to 0 because its actual value is 0.4 .4 is less than .5 so leave the number to the left of the decimal point and remove the decimal part. 15 + 0 = 15 Now take another fraction: 3 4/7: 4/7 can be rounded to 1 because its actual value is 0.5 .5 is equal to .5 so we have to add 1. 3 + 1 = 4 Now subtract: 15 – 4 = 11 Therefore, 15$\frac{3}{7}$ – 3$\frac{4}{7}$ = 11.

$McGraw Hill My Math Grade 5 Chapter 9 Lesson 9 Answer Key Estimate Sums and Differences 7$

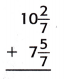
Question 13. 19$\frac{3}{7}$ + $\frac{13}{14}$ Answer: The above-given: 19 3/7 + 13/14 Now round off the fractions to the nearest whole number. 3/7 can be rounded to 0 { The actual value of 3/7 is 0.4} 13/14 can be rounded to 1 { The actual value of 13/14 is 0.9} 19 + 0 = 19 0 + 1 = 1 Now add: 19 + 1 = 20 Therefore, 19$\frac{3}{7}$ + $\frac{13}{14}$ = 20

Question 14. 7$\frac{7}{9}$ – 1$\frac{5}{18}$ Answer: The above-given: 7 7/9 – 1 5/18 Now round off the fractions to the nearest whole number. 7/9 can be rounded to 1 { The actual value of 7/9 is 0.7} 5/18 can be rounded to 0 { The actual value of 5/18 is 0.3} 7 + 1 = 8 1 + 0 = 1 Now subtract: 8 – 1 = 7 Therefore, 7$\frac{7}{9}$ – 1$\frac{5}{18}$ = 7

Question 15. $\frac{9}{16}$ + 16$\frac{5}{8}$ Answer: The above-given value: 9/16 + 16 5/8 Now round off the fractions to the nearest whole number. 9/16 can be rounded to 1 5/8 can be rounded to 1. 0 +1 = 1 16 + 1 = 17 Now add: 1 + 17 = 18 Therefore, $\frac{9}{16}$ + 16$\frac{5}{8}$ = 18

Problem Solving

Question 16. Liam spent 1$\frac{3}{4}$ hours playing board games and 2$\frac{1}{4}$ hours watching a movie. About how much time did Liam spend altogether on these two activities? Round each mixed number to the nearest whole number. Answer: The above-given: The number of hours Liam spent playing games = 1 3/4 The number of hours Liam spent watching movie = 2 1/4 The total number of hours she spent on both activities = x x = 1 3/4 + 2 1/4 Now estimate and round off the mixed fractions to the nearest whole number. 3/4 can be rounded to 1 1/4 can be rounded to 0. 1 + 1 = 2 2 + 0 = 2 Now add: x = 2 + 2 x = 4 Therefore, Liam spent 4 hours on both activities.

Question 17. Mathematical PRACTICE 2 Use Number Sense Beth walked 10$\frac{7}{8}$ miles one week. She walked 2$\frac{1}{4}$ fewer miles the following week. About how many miles did she walk the second week? Round each mixed number to the nearest whole number. Answer: The above-given: The number of miles Beth walked in one week = 10 7/8 The number of fewer miles she walked the following week = 2 1/4 The number of miles she walks = x x = 10 7/8 – 2 1/4 Now round off the mixed fractions to the nearest whole number. 7/8 can be rounded to 1 1/4 can be rounded to 0. 10 + 1 = 11 2 + 0 = 2 Now subtract: x = 11 – 2 x = 9 Therefore, she walked 9 miles.

$McGraw Hill My Math Grade 5 Chapter 9 Lesson 9 Answer Key Estimate Sums and Differences 10$

HOT Problems

Question 19. Mathematical PRACTICE 3 Justify Conclusions Select two mixed numbers whose estimated difference is 1. Justify your selection. Answer: The equation can be written as: x – y = 1 The x value would be 6 1/2 The y value would be 5 1/2 1/2 can be rounded to 1. 6 + 1 = 7 5 + 1 = 6 Now substitute and subtract: x – y = 1 7 – 6 = 1 1 = 1 Therefore, those two mixed numbers are 6 1/2 and 5 1/2.

Question 20. ? Building on the Essential Question How does number sense of fractions help when estimating sums and differences? Answer: We don’t add or subtract the denominator. When you compare fractions like 1/4 and 1/8 we have to find the factors that we represent like 4 x 2 = 8. Finally, 4 and 2 is the answer. – Estimating a fraction will give us the gist of the fraction. However, we will seldom guess the exact answer with it. If we only need a general idea of the answer, estimations are helpful.

McGraw Hill My Math Grade 5 Chapter 9 Lesson 9 My Homework Answer Key

Question 1. 2$\frac{1}{5}$ + 6$\frac{4}{5}$ Answer: The above-given: 2 1/5 + 6 4/5 Now round off the mixed fractions into the nearest whole number. 1/5 can be rounded to 0 4/5 can be rounded to 1 2 + 0 = 2 6 + 1 = 7 Now add: 2 + 7 = 9 Therefore, 2$\frac{1}{5}$ + 6$\frac{4}{5}$ = 9

Question 2. 6$\frac{5}{12}$ – 2$\frac{1}{12}$ Answer: The above-given: 6 5/12 – 2 1/12 Now round off the mixed fractions into the nearest whole number. 5/12 can be rounded to 0 1/12 can be rounded to 0. 6 + 0 = 6 2 + 0 = 2 Now subtract: 6 – 2 = 4 Therefore, 6$\frac{5}{12}$ – 2$\frac{1}{12}$ = 4

Question 3. 11$\frac{8}{9}$ + 4$\frac{1}{3}$ Answer: The above-given: 11 8/9 + 4 1/3 Now round off the mixed fractions into the nearest whole number. 8/9 can be rounded to 1 1/3 can be rounded to 0. 11 + 1 = 12 4 + 0 = 4 Now add: 12 + 4 = 16 Therefore, 11$\frac{8}{9}$ + 4$\frac{1}{3}$ = 16

Question 4. 7$\frac{1}{6}$ – 5$\frac{3}{4}$ Answer: The above-given: 7 1/6 – 5 3/4 Now round off the mixed fractions into the nearest whole number. 1/6 can be rounded to 0 3/4 can be rounded to 1 7 + 0 = 7 5 + 1 = 6 Now subtract: 7 – 6 = 1 Therefore, 7$\frac{1}{6}$ – 5$\frac{3}{4}$ = 1.

Question 5. $\frac{11}{18}$ + 6$\frac{1}{10}$ Answer: The above-given: 11/18 + 6 1/10 Now round off the mixed fractions into the nearest whole number. 11/18 can be rounded to 1 1/10 can be rounded to 0 0 + 1 = 1 6 + 0 = 6 Now add: 1 + 6 = 7 Therefore, $\frac{11}{18}$ + 6$\frac{1}{10}$ = 7

Question 6. 15$\frac{1}{3}$ – 2$\frac{5}{8}$ Answer: The above-given: 15 1/3 – 2 5/8 Now round off the mixed fractions into the nearest whole number. 1/3 can be rounded to 0. 5/8 can be rounded to 1. 15 + 0 = 15 2 + 1 = 3 Now subtract: 15 – 3 = 12 Therefore, 15$\frac{1}{3}$ – 2$\frac{5}{8}$ = 12.

For Exercises 7—9, use the pictures shown.

$McGraw Hill My Math Grade 5 Chapter 9 Lesson 9 Answer Key Estimate Sums and Differences 11$

Question 7. About how much taller is the birdhouse than the swing set? Round each mixed number to the nearest whole number. Answer: The above-given pictures: The height of the birdhouse = 14 9/16 The height of the swing set = 8 1/4 The number of feet more the birdhouse is than the swing set = x x = 14 9/16 – 8 1/4 Now round off the mixed fractions into the nearest whole number. 9/16 can be rounded to 1. 1/4 can be rounded to 0. 14 + 1 = 15 8 + 0 = 8 Now subtract 15 – 8 = 7 Therefore, the birdhouse is 7 feet more than the swing set.

Question 8. About how much taller is the birdhouse than the tree house? Round each mixed number to the nearest whole number. Answer: The above-given: The height of the birdhouse = 14 9/16 The height of the tree house = 11 13/16 The number of feet more the birdhouse is than the tree house = x x = 14 9/16 – 11 13/16 Now round off the mixed fractions into the nearest whole number. 13/16 can be rounded to 1. 9/16 can be rounded to 1. 14 + 1 = 15 11 + 1 = 12 Now subtract: x = 15 – 12 x = 3 Therefore, the birdhouse is 3 feet more than the tree house.

Question 9. Mathematical PRACTICE 1 Plan Your Solution Find the approximate height difference between the birdhouse and the tree house. Is it greater than or less than the approximate difference in height between the swing set and the tree house? Round each mixed number to the nearest whole number. Answer: The difference between a birdhouse and a tree house: The height of the birdhouse = 14 9/16 The height of the tree house = 11 13/16 The difference between birdhouse and tree house = x x = 14 9/16 – 11 13/16 Now round off the mixed fractions into the nearest whole number. 13/16 can be rounded to 1. 9/16 can be rounded to 1. 14 + 1 = 15 11 + 1 = 12 Now subtract: x = 15 – 12 x = 3 The difference between a swing set and a tree house: The height of the tree house = 11 13/16 The height of the swing set = 8 1/4 difference= x x = 11 13/16 – 8 1/4 13/16 can be rounded to 1. 1/4 can be rounded to 0. 11 + 1 = 12 8 + 0 = 8 Now subtract: x = 12 – 8 x = 4 3 < 4 Therefore, the height difference between the birdhouse and the tree house is less than the difference in height between the swing set and the tree house.

Test Practice